Some holiday Physics fun.
Problem: Two mugs of tea are poured from a boiling urn. One of them contains milk, and the other has the milk added after 5 minutes. Which one ends up cooler after 20 minutes?
Let’s make some basic (and slightly dodgy) assumptions.
- Let’s state that the milk reduces the temperature of the tea by exactly 20 degrees C, no matter when added.
- Let’s assume that the mug/cup has negligible heat capacity, and that conditions are such that the rate of heat loss is directly proportional to the temperature difference between the tea and the room.
- Let’s say the room temperature is 20 degrees C.
- Let’s guess that the liquid cools at a rate of 0.95 x temperature difference, every minute.
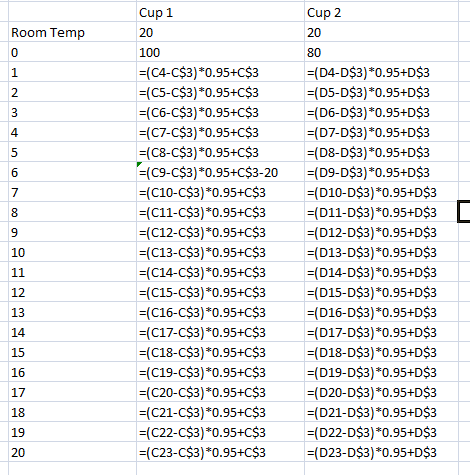
Right, let’s crack out our trusty spreadsheet. Taking my assumptions above it should be set up as:
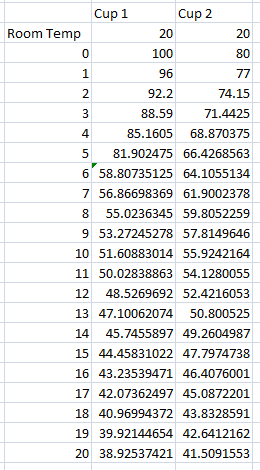
This should produce the following numbers:
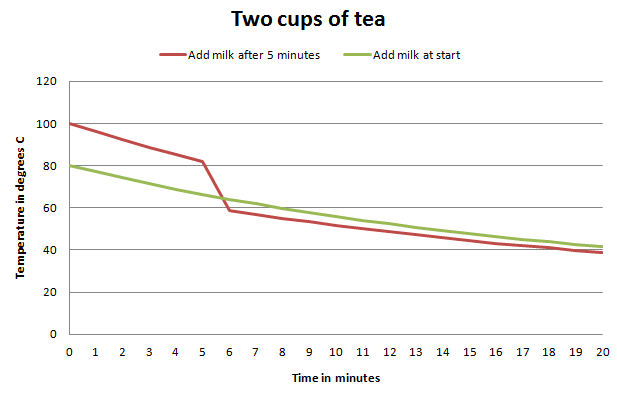
So adding after 5 minutes gets you the cooler cup! Leaving the liquid hotter for the first 5 minutes means it loses a greater quantity of heat.
Of course, there are so many other factors affecting this, you might find something a little different in practice. Plus my assumptions about milk’s cooling effect, and the effect of the mug are highly suspect. Anyone care to offer an improvement? Grab a brew and get to work!